Eighty percent of mathematical applications in everyday life require estimation rather than exact answers (Reys, 1992).
The Estimation Calculator
This is a case study that documents how one teacher uses technology to support her elementary students’ abilities to make accurate estimations. It emphasizes the TPACK framework for effective technology integration.
TABLE OF CONTENTS*
*A version of this case study appears as a chapter in the book, Practitioner’s Guide to Technology, Pedagogy, and Content Knowledge (TPACK): Rich Media Cases of Teacher Knowledge.
Introduction
The Technology, Pedagogy, and Content Knowledge (TPACK) framework is a way of describing what teachers need to know in order to effectively integrate technology in a learning experience. More than just defined, self-contained knowledge types, TPACK emphasizes an interconnected understanding of technology, best teaching practices, and subject matter expertise in order to meaningfully create technology-infused lessons.
The sections that follow highlight how an elementary teacher uses a variety of technologies to support student learning during a mathematics lesson on estimation. The interplay between the teacher’s grasp of each tool’s affordances, effective facilitation, and knowledge of the content illustrates the complex nature of TPACK in a classroom environment. Hopefully the case study will uncover the tacit actions of a master educator knows the art of teaching and maximizing student understanding.
Take some time to complete Activities 1 and 2 before beginning the case study. Both activities will help you become familiar with the Estimation Calculator, the primary technology used by students in the described lesson. Feel free to return to the Estimation Calculator at the end of Activity 2 if you want to recreate what appears during the case study.
Click the gray banners to complete each activity.
Activity 1
Watch the following screencast video tutorial on the Estimation Calculator.
Activity 2
Solve the following problem using an estimation strategy of your choosing. Enter the problem and your estimate by clicking on the Estimation Calculator button below.
444 x 222 = ?
- Did your estimate fall within the acceptable range?
- If not, how did you revise your estimate?
Scenario
Have you ever estimated the total cost of a cart full of groceries as you entered the checkout line only to be shocked by the actual bill once the cashier rang up your purchases? Computational estimation is an important skill with applications in many areas of life outside the classroom. The consequences of underestimation at the grocery story are fairly benign, but when a medical technician is unable to recognize that health equipment is generating incorrect values, patients’ lives may be at risk.
This text is a text/video-based case study that dissects a math lesson on computational estimation. It features a free, web-based application called the Estimation Calculator, a resource specifically designed to develop students’ computational estimation strategies.
Computational Estimation
Computational estimation involves making reasonable approximations for arithmetic problems, and it differs from estimations of measurement and time. Computational estimation is a form of problem solving that calls on a variety of skills used daily by adults, yet many people have never received adequate instruction on effective estimation strategies.
Teachers can begin developing children’s computational estimation abilities and expanding their range of estimation strategies in elementary school. Students are generally capable of the abstraction required for computational estimation by the late elementary grades, but explicit instruction and practice are essential.
Strong estimation abilities enhance children’s number sense, place value recognition, and understanding of the relative magnitude of rational numbers. The Common Core State Standards for School Mathematics provide guidance on children’s learning of computational estimation.
Common Core State Standards
Relevant Common Core standards for estimation include the following mathematical activities.
“Solve two-step word problems using the four operations. Represent these problems using equations with a letter standing for the unknown quantity. Assess the reasonableness of answers using mental computation and estimation strategies including rounding.”
“Solve multistep word problems posed with whole numbers and having whole-number answers using the four operations, including problems in which remainders must be interpreted. Represent these problems using equations with a letter standing for the unknown quantity. Assess the reasonableness of answers using mental computation and estimation strategies including rounding.”
Additionally, the National Council for Teachers of Mathematics (NCTM) recommends that all elementary students learn how to effectively estimate both mentally and on paper.
Estimation Strategies
Despite consensus among national organizations for flexible estimation approaches, students often learn only one strategy for estimating. A commonly employed strategy involves rounding to the largest place value without an understanding of how reasonable the estimate is in terms of the exact solution.
For example, students in the upper elementary grades often learn to estimate by rounding to the largest place value (77 x 18 becomes 80 x 20) and solve the problem (1,600). Although the answer is technically correct and the estimation strategy is valid, students rarely consider how close the estimate is to the correct solution: 1,386. Rounding to the largest place value produces an estimate that is more than 15% higher than the actual answer.
Students need to understand that other strategies may bring them closer to the actual answer. Here are some other estimation processes students can use to generate a more reasonable estimate:
TRANSLATION: Changing the operation or mathematical structure of a problem so that it is easier to estimate. For example, a translation strategy involves altering 18 + 22 + 24 to 20 x 3.
REFORMULATION: Altering the numbers in a problem so that it is easier to find an estimate. For example, a reformulation strategy involves altering 18 + 22 + 24 to 20 + 20 + 20.
COMPENSATION: Adjusting an estimate based on changes made while translating or reformulating the original problem. For example, changing 29 x 24 to 30 x 20 (reformulation) produces an estimate of 600. Compensation occurs if the student then adds 120 to the estimate of 600 to compensate for rounding 24 to 20.
What do you think?
- Which estimation processes would you choose for mental estimation and which ones for pencil and paper calculations? Why?
- How would you prompt students to try alternative estimation strategies?
Click on the button below to answer these questions. Any answer submissions will be publicly visible in the Results. Please return to this page when you finish.
Misconceptions About Estimation
In addition to being limited by their range of estimation strategies, students often possess one or more misconceptions about estimation as a result of years of focusing on algorithmic precision.
For example, students sometimes think that the exact answer is the only solution that is important. It follows, naturally, that estimation is a waste of time when emphasis is placed on exact calculations.
Students need to know the value of computational estimation before they will put effort into developing their skills. They must realize that computational estimation saves time and effort in the real world when there is not access to a calculator or when inputting information is too time consuming.
In many situations an estimate provides a useful check on a supposedly exact answer, even when using a calculator. Students should be reminded that a calculator yields a correct answer only if they enter the problem correctly. When students can appropriately estimate whether a calculator’s answer is approximately correct, they can then go on to identify possible issues related to incorrectly entering the problem.
What do you think?
- How have you helped your students develop their computational estimation skills in the past?
- How would you assess the reasonableness of students’ estimates with regards to finding an exact answer?
- What technology tools might you choose to help your students develop estimation skills?
Click on the button below to answer these questions. Any answer submissions will be publicly visible in the Results. Please return to this page when you finish.
Meet The Teacher
 Teacher: Paula White
Teacher: Paula White
Title: Gifted Research Teacher
School: Crozet Elementary School
Blog: Amplifying Minds
Twitter: @paulawhite
I am a gifted resource teacher at Crozet Elementary School in central Virginia. I have taught kindergarten through fifth grade for over forty years, both as a regular classroom teacher and gifted resource specialist. I am a Google Certified Teacher, an Apple Distinguished Educator, a STAR Discovery Educator, a Fablevision Ambassador, and a Computerworld Laureate. I also recently became a National Board Certified Teacher!
My philosophy of teaching mathematics involves a mixture of direct instruction, discourse, and hands-on activities. I think that the teacher needs to provide enough instruction so that students have a direction but not so much that the teacher is dominating the learning experience. To this end, I place great value on asking questions that elicit students’ thinking about content. Although not easy, questioning and listening are two keys to helping students move beyond a superficial understanding of mathematics in my opinion.
In Her Words: Paula White's Teaching Philosophy
With regards to teaching estimation and estimation strategies, I believe that is important for students to be able to find a range of acceptable estimates that closely approximate the correct solution. Just teaching a singular approach to estimation, like rounding to the largest place value, insufficiently prepares students to judge the reasonableness of a slew of possible estimates! Students need a repertoire of strategies and time to practice them in order to be successful estimators.
In Her Words: Paula White on Estimation Strategies
The school where I teach, Crozet Elementary School, is a small, rural school with approximately 300 students in kindergarten through fifth grade. Of these students, 24% receive free and reduced meals through federal assistance. Most of the students are White (86%); only 2.4% are African-American, 4.2% are Hispanic, and 3.5% are limited English proficient. The classroom in this case study had more females than males, which is not typical of the school in general. Of the 20 students in the class, all were classified as high performing in mathematics, with a smaller subset of the total group considered to be academically gifted. Despite the advanced characteristic of the class, I believe that this lesson is appropriate for all fifth grade students.
In Her Words: Paula White's Classroom Composition
The Lesson (According to Paula White)
Background Information
The lesson described in this case study was the third in a series of learning experiences that focused on estimation and estimation strategies. The first two lessons focused on defining estimation, evaluating when to make an estimate and when to compute an exact answer, and reviewing the estimation strategies of translation, reformulation, and compensation.
My overarching objective for this lesson was to encourage students to use alternative estimation strategies based on the reasonableness of an initial estimate. I relied on the Estimation Calculator, a free web-based application, to guide students toward increased precision, and I explained to the students that verbalizing their mathematical thinking was extremely important.
I also wanted students to have a contextual hook for why estimation was necessary, so I incorporated word problems that applied to the students’ real lives. I made informal observations of students’ conversations. At the end of the lesson, I assessed student learning formally through their written answers to questions.
The lesson occurred in three parts: Introduction, Activity, and Conclusion.
Introduction
I reviewed what the students learned in the previous two lessons using a projected computer display and a document camera. We discussed what classified as estimation before beginning two sample word problems. Seated on a rug in front of a computer projector, students worked in pairs to solve the following problems as I circulated and assisted.
1. Crozet Elementary fifth grade classes had a food drive for the Blue Ridge Area Food Bank. About how much was collected in all?
- Ms. W’s class collected 346 pounds.
- Ms. X’s class collected 402 pounds.
- Ms. Y’s class collected 388 pounds.
- Ms. Z’s class collected 327 pounds.
2. Eight people enter an elevator. The approximate weight of each person is 176 pounds.
- About how much weight is on the elevator?
- Is this over or under the maximum weight allowed (1,500 lbs)?
I went over the problems when the students finished, listened to the estimates they derived, and prompted them to explain their estimation strategies as they showed their work beneath the document camera. I then explained that they would be using the Estimation Calculator.
TPACK Commentary
Connecting previously learned content to the current lesson objectives creates a narrative arc that ties learning experiences together. Reviewing past lessons also enables the teacher to assess whether or not students grasp underlying concepts that are necessary for more complex tasks. In this case, Paula chose to use a projector and a document camera to review past work and the sample word problems. The document camera allowed students to share their work with the class in a convenient and easy way. Her decision to use these technologies related to the usefulness of the technologies.
Activity
The students began the activity by watching a screencast video about how to use the Estimation Calculator with a partner. The screencast was displayed on an iPod Touch®, and the students listened on shared earbuds as they worked through a sample problem.
See It Happen
Once finished, student pairs solved four problems designed to encourage use of multiple estimation strategies. The four problems were as follows.
Estimate the answer to each one of these problems. Show your work on paper and then check your answer using the Estimation Calculator. Work with your partner!
- 149 + 338
- 449 + 144
- 77 x 18
- 26 x 59
For each problem, one partner was assigned the role of “worker,” and this person made an estimate and verbalized the strategy that was used. The other student’s role was to take notes and write down the worker’s strategy.
Once an estimate was made and the strategy was recorded, the worker entered the problem and the estimate into the Estimation Calculator. If the estimate was greater than 15% above or below the exact answer, then the worker revised the estimate using a different estimation strategy. The students switched roles and moved to the next problem when a reasonable estimate was made.
What do you think?
- What might be the benefits of having students watch a screencast on a small, portable media player while learning how to use a new technological tool?
- What technology troubleshooting might be required when students use a tool like this?
- How does Paula use student roles to maximize student participation in the activity?
Click on the button below to answer these questions. Any answer submissions will be publicly visible in the Results. Please return to this page when you finish.
Conclusion
The lesson’s conclusion incorporated a synchronous, online discussion using a tool called TodaysMeet. I asked the students to enter responses to a question: “Is it better to overestimate or underestimate?” I then encouraged students to explain their reasoning with real world examples.
See It Happen
TPACK Commentary
Paula deliberately chose to use a synchronous discussion tool to examine students’ thoughts on the concluding question. Writing answers to questions instead of raising hands and talking out loud often encourages more participation from students who are typically silent during large group conversations. Furthermore, synchronous online discussion tools enable all participants to engage in simultaneous dialogue whereas typical whole class discussions feature fewer voices.
Primary Technology: The Estimation Calculator
In this lesson, Paula chose to use the Estimation Calculator, a software application developed specifically to develop students’ skills in computational estimation, estimation strategies, and making reasonable estimates.
The Estimation Calculator provides a visual indicator of the reasonableness of an estimate within a certain percentage above or below the exact answer. Paula used this tool to encourage students to implement different estimation strategies for addition and multiplication problems involving whole numbers. The Estimation Calculator provides feedback on the reasonableness of estimates to mathematical problems.
Users enter an addition, subtraction, multiplication, or division problem as well as an estimated solution, and the Estimation Calculator displays a visual indicator that shows how close the estimate is to the correct answer. If the estimate is within +/- 15% of the answer, then the Estimation Calculator will display the correct solution. Users are prompted to try again if the estimate is outside of the range.
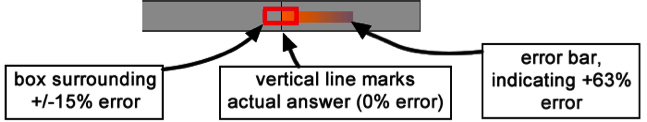
Visual feedback is an important component within the Estimation Calculator (see image above). A red box provides the parameters for the range of acceptable estimates, and an orange bar indicates how close the estimated solution is to the correct answer. These indicators prompt students to use alternative estimation strategies in an attempt to find a better approximation.
In His Words: Robert Q. Berry III on The Estimation Calculator
Robert Q. Berry III is an Associate Professor of Mathematics at the University of Virginia. He co-created the Estimation Calculator with Peter Malcolm.
Using the Estimation Calculator to provide feedback for making reasonable estimates aligns with the following ISTE National Educational Technology Standards for Students (NETS-S):
RESEARCH & INFORMATION FLUENCY: Students apply digital tools to gather, evaluate, and use information.
CRITICAL THINKING, PROBLEM SOLVING, & DECISION MAKING: Students collect and analyze data to identify solutions and/or make informed decisions.
What do you think?
- If you could create the ideal tool for helping students develop their computational estimation skills, what features would it have?
- What technological preparation would students need before using the Estimation Calculator most effectively?
- What classroom management strategies would you use to keep students on task with this assignment?
- How might you use this technology with a class of students who are not high performing in mathematics?
Click on the button below to answer these questions. Any answer submissions will be publicly visible in the Results. Please return to this page when you finish.
Classroom In Action
Throughout the activity portion of the lesson, receiving feedback on the reasonableness of an estimate became an impetus for students’ use of various estimation strategies.
Vignette 1
Watch the following video clip and look for instances of estimation strategies and revisions. Pay attention to what Paula says as well as the students’ verbal interactions before and after using the Estimation Calculator.
What do you think?
- What estimation strategies (Reformulation, Translation, and/or Compensation) did the student used to arrive at the initial and final estimate? Why?
- What kinds of open-ended questions did Paula ask to encourage the student to reconsider her estimate?
- How did the Estimation Calculator facilitate changes in the student’s thinking about a reasonable estimate?
Click on the button below to answer these questions. Any answer submissions will be publicly visible in the Results. Please return to this page when you finish.
Vignette 2
Watch the following video clip and pay attention to how Paula elicited the student’s estimation strategy. Think about what might have happened if Paula did not ask the student to explain her estimation process.
What do you think?
- What estimation strategies (Reformulation, Translation, and/or Compensation) did the student use to solve the problem? Why?
- How did Paula incorporate mathematical language like “compatible numbers?”
- What role did the Estimation Calculator play in terms of evaluating the reasonableness of the student’s estimate?
- In both Vignette 1 and Vignette 2, what evidence did you observe that students were learning and developing their estimation skills?
Click on the button below to answer these questions. Any answer submissions will be publicly visible in the Results. Please return to this page when you finish.
TPACK Commentary
Students often search for the correct solution when working math problems. Arriving at the answer is sometimes seen as the goal, and the mathematical thinking that underlies the solution goes unnoticed without teacher guidance and prompting. However, when a skilled educator prods and prompts students to explain their thinking, insights into both students’ conceptual understanding and their misconceptions are revealed. In the Vignette 1, Paula uses the visual feedback bar in the Estimation Calculator to prompt the girl to revise her strategy. The questions that she asks are broad but guiding in that they facilitate more precise rounding. In the Vignette 2, Paula simply asks the student to explain her thinking so that she knows the estimation strategy that was used.
Student Work
Assessing Written Answers (According to Paula White)
Students were scattered throughout the room during the activity portion of the lesson. Some were sitting on the floor beneath the whiteboard, others at desks, and a few lounging on the classroom couch.
As I walked around and observed, I saw that most students were busily discussing their estimation strategies with their partner. I immediately noticed that students were having rich discussions about the problems. They were checking their estimates on the Estimation Calculator and, in many cases, revising their strategies. However, it was quite apparent that the conversations did not correlate with what was being written on their worksheets.
For example, one pair had a rich dialogue about how to estimate 26 x 59. The student making the estimate for this problem explained that rounding to the tens place for both numbers (30 x 60) produced an estimate of 1,800, which was outside of the range of a reasonable approximation based on the Estimation Calculator. The student then rounded 26 to 25 and explained that rounding down would produce a more accurate estimate because the rounded number was closer to the original number.
When I looked at the written account of this pair’s estimation strategies, I immediately saw that the original attempt to estimate was not documented nor was the explanation about why 25 was a better approximation of 26. Had I not witnessed the conversation, I would have never known that these students were wrestling with alternative strategies.

I reviewed all of the worksheets and saw a lack of written clarity and specificity much like the previous example. It was almost as if the conversations were too complex and too long for the students to clearly capture in the space provided. Yet, I wanted the students working in pairs and talking to each other because I believed that verbally sharing encouraged students to expand their repertoire of strategies and approaches. In my opinion, mathematical discourse was a powerful way to strengthen conceptual knowledge of estimation.
I would consider audio recording the conversations in the future instead of having them write the dialogue on a worksheet. For me, understanding thought processes is more important then having a tangible, written artifact. I also think that audio recording would provide the class with opportunities to hear other individual’s estimation strategies. Yet, recording potentially creates more overhead in terms of saving files and instructing students on how to use an audio recorder.
What do you think?
- How would you address the issue of students insufficiently describing their thought processes on paper?
- How would you assess students’ learning growth after this activity?
Click on the button below to answer these questions. Any answer submissions will be publicly visible in the Results. Please return to this page when you finish.
Cumulative Assessment (According to Paula White)
I gave all of the students a short written assessment on the day after the lesson. The assessment included questions for the upcoming unit as well as two questions pertaining to estimation.
The first estimation question was a 4-digit by 4-digit subtraction problem, and the second question was a 2-digit by 2-digit multiplication problem. I asked the students to estimate the answer and described the estimation strategy that they used to arrive at the approximation. Students wrote their answers on paper and did not use the Estimation Calculator to check for reasonableness. I chose not to use the Estimation Calculator in the assessment because I wanted to see whether or not students would use alternative strategies that did not involve rounding to the largest place value without being prompted by the tool’s visual feedback.
The results were quite interesting! All of the students used a variety of strategies to solve both of the problems even when not using the Estimation Calculator. The subtraction problem produced the most diverse set of answers (9,644 – 5,788). One student articulated that he initially rounded both numbers to the hundreds place (9,600 – 5,800). He then realized that the rounded numbers were not easy to mentally subtract, so he rounded 9,644 to 10,000 and then subtracted 5,800 from 10,000 to arrive at his estimate (4,200).
Although rounding to the largest place value would have resulted in a reasonable estimate on the Estimation Calculator had it been used, this student chose to use a more precise estimation strategy that involved compatible numbers. I am fairly certain that this student would not have answered the question in the same way had he not practiced problems using the Estimation Calculator.
Students also wrote clearer descriptions of their strategies on the assessment than what was shown on the worksheets during the lesson. There was much more detail, and I could clearly follow the strategy that was used to arrive at the estimate. I am unsure if the results are a reflection of the fact that the questions appeared in an assessment or if it was because they were working individually. Regardless, I was pleased to see that the students were able to articulate an estimation strategy in clearly.
What do you think?
- How would you improve this activity?
- In what ways could the learning goals be approached in a different way?
Click on the button below to answer these questions. Any answer submissions will be publicly visible in the Results. Please return to this page when you finish.
Teacher Reflection
Estimation Strategies
I honestly think that incorporating the Estimation Calculator into my estimation lessons forced students to think about using different strategies.
Students were less reliant on basic rounding and more adept at examining the original numbers as clues for creating an estimate after the lesson. I believe that this change was a result of the visual feedback that the Estimation Calculator provided.
Even though some were quite good at finding an arithmetically correct estimate, seeing how far away the estimate was from the exact answer made students reconsider both their answer as well as the process for achieving the solution. Students internalized the need to make more precise estimates, and many demonstrated this on problems that did not include the Estimation Calculator.
In Her Words: Paula White on The Estimation Calculator
Why The Lesson Worked
Two key components in this lesson’s success were paired collaboration and probing questions.
Students listened to their partner’s estimation strategies and, in many instances, offered their personal approach without being prompted. This was especially true when the Estimation Calculator showed that an estimate was unreasonable and outside of the 15% range. The exchange of ideas in a one-on-one, student-to-student situation enhanced the applicability of various strategies in ways that a more teacher-centered, directive manner would fail to achieve.
Yet, I was able to intercede and ask open-ended questions when conversations either stalled or lacked a full explanation. I even overheard students asking for clarification. Asking questions was very important because I wanted to understand how each child was thinking mathematically.
In Her Words: Paula White on Student Thinking
The Technology
I did not experience any major classroom management issues with the technology. I am certain that this was a result of the cumulative experiences that the students have using various tools on a daily basis. The students knew my expectations of their technology use, and they acted accordingly.
However, that does not mean that everything went smoothly!
One problem that became quite apparent was that the sound quality on the iPod units was very poor when played through the built-in speakers. Students could not hear what was being said in the video when they were learning how to use the Estimation Calculator. Luckily the students adapted by grabbing a pair of headphones and sharing the earbuds. When I teach the lesson again, I will make sure to include headphones or show the video on individual computers.
In Her Words: Paula White on Classroom Technology Use
What do you think?
- Consider your classroom. What potential classroom management issues exist that might prevent you from successfully incorporating a tool like the Estimation Calculator?
Click on the button below to answer this question. Any answer submissions will be publicly visible in the Results. Please return to this page when you finish.
Notes
The content in this case study appears as a chapter in the following edited book. For the final published version as well as other technology case studies, please visit AACE’s EditLib.
Kjellstrom, W., Berry, R. Q., & White, P. (2015). Elementary Math. In Hofer, M., Bell, L. & Bull, G. (Eds.), Practitioner’s Guide to Technology, Pedagogy, and Content Knowledge (TPACK): Rich Media Cases of Teacher Knowledge (pp. 1-14). Waynesville, North Carolina: Association for the Advancement of Computing in Education (AACE).
References
COMMON CORE STANDARDS
© Copyright 2010. National Governors Association Center for Best Practices and Council of Chief State School Officers. All rights reserved. Retrieved January 17, 2016, from http://www.corestandards.org/public-license/
ESTIMATION STATISTIC
Reys, Barbara J. “Estimation.” In Teaching Mathematics in Grades K-8: Research-based Methods, edited by Thomas Post, 279-301. Boston: Allyn & Bacon, 1992.
ISTE STANDARDS
ISTE Standards for Students. (2014, August 5). Retrieved January 17, 2016, from http://www.iste.org/standards/ISTE-standards/standards-for-students

